SOLUTION
Part A
Equation of a circle is given as
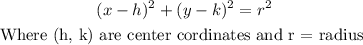
So for circle A, center (0, 0) and radius 6, the equation of the circle is
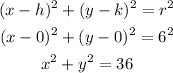
For circle B, center (-4, -2) and radius 4, the equation of the circle is
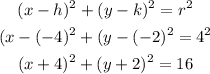
Making the graphs, we have
So the large green circle is for A and the smaller Blue circle is for B
Part B
The sequence of transformations that maps circle B unto circle A
We can see that circle B is smaller than circle A, that means circle B was derived from circle A being shrinked.
Now, from their radii, circle A is 6 units and circle B is 4 units. So the scale factor is
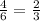
So, the transformation a dilation; circle A was shrinked by a scale factor less than 1.
Also We can see that a shift was noticed. 4 units to the left and 2 units down.
Hence the answer is
The transformation is a dilation; circle A was shrinked by a scale factor less than 1 and was shifted 4 units to the left and 2 units down.