Answers:
a) midpoint: (1, 4)
b) slope of PQ: 1/3
c) length of PQ: √40
d) equation of perpendicular bisector of PQ:
Step-by-step explanation:
The given points are P(4, 5) and Q(-2, 3)
Part a)
The midpoint of two points (x1, y1) and (x2, y2) can be calculated as

So, replacing (x1, y1) = P(4, 5) and (x2, y2) = Q(-2, 3), we get:
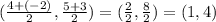
Then, the midpoint of PQ is (1, 4)
Part b)
The slope of a segment that passes through points (x1, y1) and (x2, y2) can be calculated as
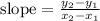
Replacing (x1, y1) = P(4, 5) and (x2, y2) = Q(-2, 3), we get:
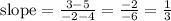
So, the slope is 1/3
Part c)
The length of a segment that goes from (x1, y1) to (x2, y2) is calculated as
![\sqrt[]{(x_2-x_1)^2+(y_2-y_1)^2}](https://img.qammunity.org/2023/formulas/mathematics/college/s91s233vun4sfi433g8exahmp5kohnyvcn.png)
Replacing (x1, y1) = P(4, 5) and (x2, y2) = Q(-2, 3), we get:
![\begin{gathered} \sqrt[]{(-2-4)^2+(3-5)^2} \\ \sqrt[]{(-6_{})^2+(-2)^2} \\ \sqrt[]{36+4} \\ \sqrt[]{40} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/p4thnhebbk7748q3luwc9opbxqtw800dqn.png)
Therefore, the length of PQ is √40.
Part d)
The perpendicular bisector of PQ is a line that divides the segment into two equal and forms a 90 degrees angle with the segment.
First, we need to calculate the slope of the line. Taking into account that the slope of perpendicular lines multiply to -1, we get that the slope of the perpendicular bisector is
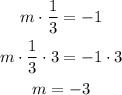
Additionally, the perpendicular bisector will pass through the midpoint of PQ, so it will pass through (1, 4).
Now, the equation of a line with slope m= -3 that passes through the point (x1, y1) = (1, 4) is

Therefore, the equation of the perpendicular bisector is y = -3x + 7.