Question

Determine which equation is parallel to line BC and which is perpendicular to line BC. YA +10 -8 -6 -4 С. +2 -10 -8 -6 4 -2 2 4 6 00 10 -2 -4 --6 -8 -10 Parallel Line Perpendicular Line

Answer 1

1) Considering that BC has 2 points (4,2) and (-5,-4) let's find the slope

`m=(-4-2)/(-5-4)=(-6)/(-9)=(2)/(3)`

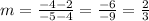
Let's find the linear coefficient "b" in the y=mx+b plugging point (4,2) into that

y= 2/3x +b

2 =2/3(4) +b

2=8/3 + b

2-8/3=b

-2/3=b

y= 2/3x -2/3 The line BC is described by this equation

Rewriting it into the Standard form

y=2/3x -2/3

-2/3x +y=-2/3 Multiply by 3

-2x + 3y = -2

2x -3y = 2

2) Parallel lines have the same slope and Perpendicular lines have reciprocal and opposite slopes, in this case, the perpendicular slope must be -1/m slope. so examining the options we have

a) 4x -6y = 7 /2 (y=2/3x -7/6)

2x -3y = 7/2 Parallel line

Then we have a parallel line because the slope is the same and proportional

b) 4x +6y=3 / 2 (y=-2/3x +1/2)

2x +3y = 3/2 Not parallel, not perpendicular

c) 3x +2y=5 (y=-3/2x+5/2) Perpendicular

d) 3x- 2y=1 (y=3/2x-1}{2}