Question

Because of the long time of the session,the tutor was unable to solve the last 3 steps,so you need to continue it, Points 1 to 6To present y=-x^3+3x^2, 9 points must beselected, point1: Domain,2: zeros of the function,3: period and symmetry,4: sign of the function,5: going to the edge of the function,6: asymptotes,7: monotony and extreme values,8: concavity and convexity,9: to present the function graphically.

Answer 1

Given that

The equation is

`y=-x^3+3x^2`

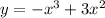
Explanation -

So we have to find points 7, 8, and 9 for the given equation.

7. Monotony and Extreme values -

Monotony is checked by finding the derivative of that function and extreme values are also found by equating the derivative of f(x) equals to zero.

`\begin{gathered} y=f(x)=-x^3+3x^2 \\ \\ So \\ f^(\prime)(x)=-3x^2+6x \\ \\ On\text{ equating it equal to zero } \\ -3x^2+6x=0 \\ -x(3x-6)=0 \\ x=0\text{ and 3x-6=0} \\ x=0\text{ and }x=(6)/(3)=2 \\ x=0\text{ and }x=2 \end{gathered}`

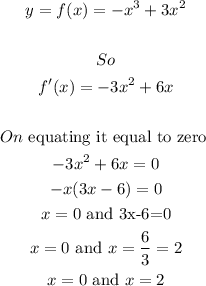
So the extreme values are 0 and 2.

8. Concavity and convexity -

To find these values we have to find the second derivative of the function and then solve it if the answer is positive it is convex and if it is negative it is concave.

`\begin{gathered} f(x)=-x^3+3x^2 \\ f^(\prime)(x)=-3x^2+6x \\ f^(\prime)^(\prime)(x)=-6x+6 \\ \\ On\text{ substituting the values we have} \\ \\ -6x+6=0 \\ 6x=6 \\ x=(6)/(6)=1 \\ x=1 \\ So\text{ the function is convex.} \end{gathered}`

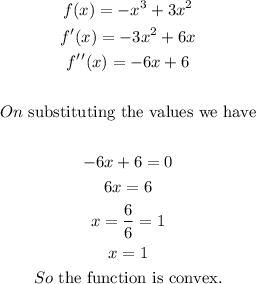
Hence the convexity is 1.

9. The graph of the function is as

Final answer -

The answers are 7. 0 and 28. Convexity is 1.9. Graph is attached above.