Question

For ach line find the slope between the 2 points given - simplify each fraction to prove that the line have a CONSTANT rate of change :1) Point A :2) Point B : 3) Point C : 1) Slope of AB :2) Slope of BC :3) Slope of AC :Describe the SLOPE of the line :Therefore the CONSTANT rate of change it....?

Answer 1

EXPLANATION

As we already know, the slope formula will be as follow:

`\text{Slope}=((y_2-y_1))/((x_2-x_1))`

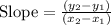
Let's consider:

Point A: (x1,y1)= (-5,5) and Point B: (x2,y2)=(-2,2)

Replacing these two ordered pais in slope formula:

`\text{Slope}=\frac{rise}{\text{run}}=((2-5))/((-2-(-5)))=(-3)/(3)=-1`

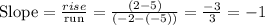
The slope of AB is -1.

Therefore, as BC and AC is a part of the same line trend, the slope of both ordered pairs will be the same.

1) Slope of AB : -1

2) Slope of BC :-1

3) Slope of AC :-1

Describe the Slope of the line:

This slope is negative and the line is downwards.