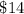Given that:
- On the first day the school sold 5 senior citizen tickets and 3 student tickets for a total of $92.
- On the second day the school took in $72 by selling 3 senior citizen tickets and 3 student tickets.
Let be "x" the price (in dollars) of a senior citizen and "y" the price (in dollars) of a student ticket.
Using the data provided in the exercise, you can set up this System of Equations:
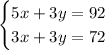
You can solve it using the Elimination Method:
1. Multiply the first equation by -1.
2. Add the equations.
Then:
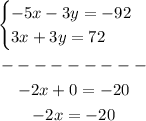
3. Solve for "x":
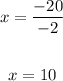
4. Substitute the value of "x" into one of the original equations and solve for "y":
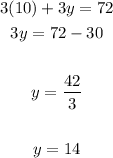
Hence, the answer is:
- Price of a senior citizen ticket:
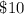
- Price of a student ticket: