ANSWER
The system has no solution
Step-by-step explanation
We want to find the solution to the system of equations given:
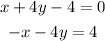
From the first equation, make x the subject of the formula:
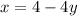
Substitute that into the second equation and simplify:
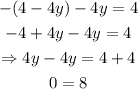
As we can see, the two sides of the equality sign have two different values.
This implies that the system of equations has no solution.