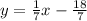Given:
The coordinates of the points through which the line passes,
(x1, y1)=(-3, -3).
(x2, y2)=(4, -2).
The two point form of the equation of a line can be expressed as,
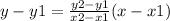
Substitute the known values in the above equation.
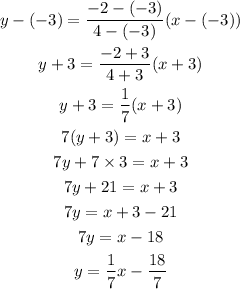
Therefore, the equation of the line is,