Question

Identify the parent function of the function g (x) = (x-8) ^ 3 from its function rule. Then graph g and identify what the transformation of the parent function it represents.

Answer 1

The parent function of the function
`g (x) = (x-8) ^ 3` and the transformation of the parent function it represents is: B. cubic; translation rigth 8 units.

In Mathematics and Geometry, the translation or horizontal shift of a graph to the right would add a number to the numerical value on the x-coordinate of the pre-image:

g(x) = f(x - h)

Based on the information provided, we can logically deduce that the transformed function
`g (x) = (x-8) ^ 3` was produced by horizontally shifting a parent cubic function
`f(x)=x^3` based on the exponent of 3;

`g(x) = f(x -h)^3\\\\g(x) = (x -8)^3`

Answer 2

Given:

The function

`g(x)=(x-8)^3`

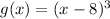
Required:

Graph g and identify what the transformation of the parent function it represents.

Step-by-step explanation:

The graph of given function and parent function:

So, the parent function is cubic and it is translated 8 units to right.

Answer:

Second option is correct.