The function is continuous if f(c) is defined, where c is a constant and the limit of x approach to c give the same value of f(c)
For the given function

If f(-3) is defined, then the function is continuous at this value
Let us find f(-3) by substituting x by -3
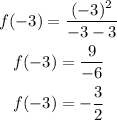
Then f(-3) = -3/2
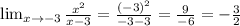
Since f(-3) has the same value of the limit of x approach to -3, then
The function is continuous at x = -3