Question

This is a practice assessment not for a grade! I just need help understanding it and the answer to be able to have an example to look back to

Answer 1

`\angle MP=64^0`

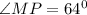
And it is the angle at the centre of a circle

The theorem,

Angle at the centre of a circle = 2 x angle at the other part of the circumference

Hence;

`\begin{gathered} \angle MP\text{ = 2 x }\angle N \\ 64^0=2\text{ x }\angle N \end{gathered}`

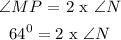
Divide both sides by 2

`\begin{gathered} \angle N=(64)/(2) \\ \angle N=32^0 \end{gathered}`

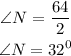
Part B:

We are to find the angle at the centre in this case

The angle at the centre is NQ and P is the angle at the circumference

Applying the same theorem as we used in part A, that is

Angle at the centre of a circle = 2 x angle at the other part of the circumference

`\begin{gathered} \angle NQ\text{ = 2 x }\angle P \\ \angle NQ\text{ = 2 x 53} \\ \angle NQ=106^0 \end{gathered}`

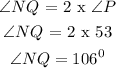
Hence, angle N = 32 degrees and angle NQ = 106 degrees.