The cosine of the angle is -5/13 given that
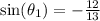 and in quadrant III
and in quadrant III
How to determine the cosine of the angle
From the question, we have the following parameters that can be used in our computation:
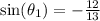
The cosine of the angle can be calculated using the following equation
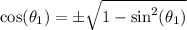
Substitute the known values into the equation
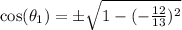
So, we have
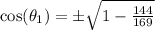
This gives
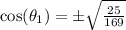
So, we have
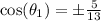
Cosine is negative in the third quadrant
So, we have
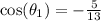
Hence, the cosine of the angle is -5/13