Linear functions
They can be identified because their expression has the form:
y= f(x) = mx + b
Where m is the slope of the line and b is the y-intercept
To define a line, we only need two points. If we have more than two points, we can test if the rest of them belongs to the line.
Let's take two consecutive points of the table and calculate the slope. If the slope is constant through all the tables then the function is linear.
Table A
Points (-3,6) (-1,2)
Slope:
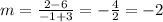
Points (-1,2) (1,-2)
Since all the slopes are equal, this is a linear function
Note the slope is different, thus this is not a line
The slope is not constant, thus this is not a line
The slope is not constant, thus this table is not a linear function