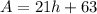Question

Enter the missing values in the area model to find 7(3h+9)

Answer 1

The are of a rectangle is:

`A=b\cdot h`

b= base

h= heigh

As you have in the first rectangle the base (3h) and the heigh(7), you find the area:

`A=7\cdot3h=21h`

In the second rectangle you have the area (63) and the heigh (7), you fin the base:

`\begin{gathered} A=b\cdot h \\ \\ b=(A)/(h) \\ b=(63)/(7)=9 \end{gathered}`

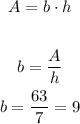
You get:

The total base of the rectangle is:

`b=3h+9`

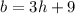
Then the total area is:

`\begin{gathered} A=7\cdot(3h+9) \\ \end{gathered}`

Or the add of the two areas:

`A=21h+63`