Question

Solve the following system of equations. State whether the system is consistent and independent, consistent and dependent, or inconsistent.–4x + 7y = 284x – 7y = 32 1) consistent and independent2) consistent and dependent3) inconsistent

Answer 1

Here we have the following system of equations:

`\begin{cases}-4x+7y=28 \\ 4x-7y=32\end{cases}`

We could solve it by the substitution method.

We're going to solve any of both equations given for a variable. I'll pick the variable "y" and the equation 1.

`\begin{gathered} -4x+7y=28 \\ 7y=28+4x \\ y=4+(4)/(7)x \end{gathered}`

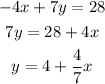
We're going to replace the previous expression in the equation 2. This is:

`\begin{gathered} 4x-7(4+(4)/(7)x)=32 \\ 4x-28-4x=32 \\ -28\\e32 \end{gathered}`

As you can see, the system is inconsistent. So it doesn't have a solution.