Solution
(a) setting f(x) = 0

(b)
when x = 0
y = f(0) = -108
Hence, the height is 108
(c)
Maximum = (1.5, 15.188)
(d)
Minimum (-1.702, -300) and (4.702, -300)
(e) From the graph, the interval of increasing is

(f) From the graph, the interval of decreasing is;
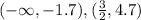
g)