You have to calculate the interest Betty will earn after three years, if she has an initial amount of $134 and the account compounds annually at a 2% interest rate.
To determine the total interest after three years, the first step is to calculate the accrued amount. We know that the accrued amount after a t period of times is equal to the principal amount (or initial amount) plus the interest
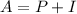
A= accrued amount
P= principal amount
I= interest
To calculate the accrued amount of an account with compound interest you have to use the following formula:

Where
A= accrued amount
P= principal amount
r= interest rate expressed as a decimal value
t= time period involved, expressed as years
n= number of compounding periods per unit of t
Compounding periods
This account compounds annually, which means that there is one compound period per year, If the time is 3 years, the total number of compounding periods will be:
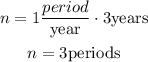
To express the interest rate as a decimal value you have to divide the percentage by 100:
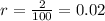
The principal amount is P=134, and the time period is t=3, so the accrued amount can be calculated as:
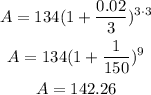
The accrued amount after 3 years will be $142.26
To determine the interest you have to subtract the principal amount from the accrued amount:
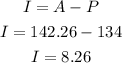
So the total interest after 3 years will be I=$8.26