Question

A 4 foot hiker casts a 8-foot shadow, and a nearby tree casts a 27-foot shadow.To the nearest tenth, the height of the tree is

Answer 1

Given:

Height of hiker = 4 feet

Length of hiker's shadow = 8 feet

Length of tree's shadow = 27 feet

Let's find the height of the tree.

We have:

4 feet = 8 feet

x feet = 27 feet

`(4)/(8)=(x)/(27)`

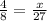
Where x represents the height of the tree.

Let's solve for x.

Cross multiply:

`\begin{gathered} 8(x)=27(4) \\ \\ 8x=108 \\ \\ \text{Divide both terms by 8:} \\ (8x)/(8)=(108)/(8) \\ \\ x=13.5 \end{gathered}`

Therefore, the height of the tree is 13.5 feet

ANSWER:

13.5 feet