Question

a right triangle is shown below with an altitude drawn from the right triangle to the hypotenuse. Prove that triangles ABC and BDC are similar!

Answer 1

A right triangle is given with its altitude drawn from the right angle to its hypotenuse.

It is required to prove that the triangles ABC, ADB, and BDC are similar.

Recall the Angle-Angle Similarity (AA~) Theorem:

If two angles of one triangle and two corresponding angles of another triangle are congruent, then the triangles are similar.

Consider the triangled ABC and ADB:

By the reflexive property of angle congruence, it follows that:

`\angle A\cong\angle A`

Since the angles B and D are right angles, from the right angles congruence theorem, it also follows that:

`\angle B\cong\angle D`

Hence, by the Angle-Angle Similarity (AA~) Theorem, it follows that triangles ABC and ADB are similar.

Consider triangles ABC and BDC:

By the reflexive property of angle congruence, it follows that:

`\angle C\cong\angle C`

Since the angles B and D are right angles, from the right angles congruence theorem, it also follows that:

`\angle B\cong\angle D`

Hence, by the Angle-Angle Similarity (AA~) Theorem, it follows that triangles ABC and BDC are similar.

We now have:

`\triangle ABC\cong\triangle ADB\text{ and }\triangle ABC\cong\triangle BDC`

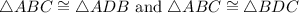
By the transitive property of similarity, it follows that:

`\triangle ABC\cong\triangle ADB\cong\triangle BDC`

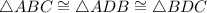
Hence, the three triangles are all similar.