Answer:
135751 unique four card combinations can be created
Explanations:
Total number of cards in a deck = 52
Number of queens in a deck = 4
Number of jacks in a deck = 4
If all the queens and jacks are removed, number of remaining cards = 52 - 8
Number of remaining cards = 44
Number of unique four card combinations that can be created = 44C4
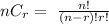
Therefore:
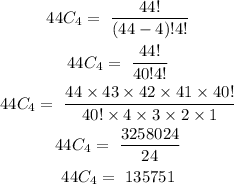
135751 unique four card combinations can be created