Answer:
P = 0.0233t - 0.0633
Explanations:
The standard linear equation in point-slope form is expressed as;
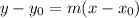
where:
• m is the ,slope, of the equation
,
• (x0, y0) is ,any point ,on the line
Let t be the number of years
Let P be the percentage of soldiers unavailable
This can be written in the coordinate form (t, P)
If the percentage of soldiers who are unavailable for combat has increased approximately linear from 10% in 2007 to 17% in 2010, the coordinate points on the line will be (7, 0.10) and (10, 0.17)
Determine the slope of the equation:
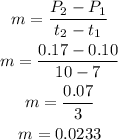
Substitute any of the points and the slope into the expression below:

Simplify to get the required linear equation:
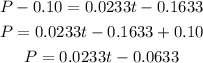
Hence the equation of a linear model to describe the data is P = 0.0233t - 0.0633