Answer:
30 minutes
Step-by-step explanation:
We were given the following information:
The tank can hold 5.400 liters of water
Two pipes can be used to fill the tank:
The first tank alone can fill the tank in 90 minutes
The second tank alone can fill the tank in 45 minutes
We thus have:
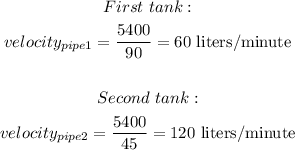
For the combined flow of both pipes, we have:

Therefore, the tank will be filled in 30 minutes