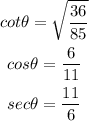Given:
A triangle is given as below
Find:
we have to find the values of cotangent, cosine and secant at theta.
Step-by-step explanation:
we know,

since, angle is less than 90 degree, so we will take only positive value of cotangent,
Therefore, the required values are given as below