Given:
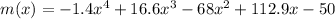
m(x) represents Mariana's design while the given graph represents Chloe's design.
Here, x is the horizontal distance from the left edge of the design.
Let's find the design which has the lowest valley floor over the first 6 inches.
From the graph, the lowest valley for Chloes's design c(x) is approximately 9.5 inches.
For Mariana's design, we have the graph below:
From the graph above, we can see that for m(x), there is no valley for the first 6 inches.
Therefore, we can say Marianna's design doesn't have a valley floor for the first 6 inches of the design.
• ANSWER:
Marianna's design doesn't have a valley floor for the first 6 inches of the design.