Given,
The initial length of the lead pipe, L=50 m
The initial temperature, T₁=16 °C=289.15 K
The temperature of the pipe when the hot water flows through it, T₂=80 °C=353.15 K
The coefficient of linear expansion of the lead is α=29×10⁻⁶ K⁻¹
The expansion in the length of the pipe is given by,
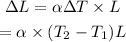
On substituting the known values,
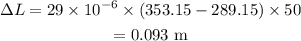
Therefore the pipe expands by a length of 0.093 m
Thus the new length of the pipe is
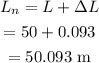
Thus the length of the hot pipe is 50.093 m