Question

7. Write the inverse of y = Vx + 10 given x > -10

Answer 1

To obtain the inverse of the function, the following steps are necessary:

Step 1: Make x the subject of the formula in the equation that represents the function, as follows:

`\begin{gathered} \text{Given: y= }\sqrt[]{x+10} \\ \text{Square both sides: y}^2=(\sqrt[]{x+10})^2 \\ \Rightarrow y^2=x+10 \\ \text{subtract 10 from both sides} \\ y^2-10=x+10-10 \\ \Rightarrow y^2-10=x \\ \text{Thus:} \\ x=y^2-10 \end{gathered}`

Step 2: Now, let x and y swap places, as follows:

`\begin{gathered} From\colon x=y^2-10 \\ it\text{ becomes:} \\ y=x^2-10 \end{gathered}`

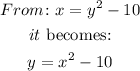
Therefore:

`\begin{gathered} \text{the inverse of : y= }\sqrt[]{x+10} \\ is\text{ : y}=x^2-10 \end{gathered}`

The answer is: y = x^2 - 10