Question

Find the angle between the following two lines: 2x + 3y = 5x − 3y = 6 (round your answer to the nearest tenth of a degree).

Answer 1

In order to find the angle between the lines, first solve each given equation for y, as follow:

2x + 3y = 5 subtract 2x both sides

3y = -2x + 5 divide by 3 both sides

y = -2/3 x + 5/3

x - 3y = 6 subtract x both sides

- 3y = -x + 6 divide by -3 both sides

y = 1/3 x - 2

next, take into account that the general equation of a line can be written as follow:

y = mx + b

where m is the slope and b the y-intercept.

Consider that the tangent of the inclination angle of the line is equal to the slope of the line.

You can notice that the slopes of the lines are m1 = -2/3 and m2 = 1/3 respectively.

Then, you have:

tanθ1 = -2/3

tanθ2 = 1/3

apply inverse tangent to the previous equations to get the values of the angles:

`\begin{gathered} \theta_1=\tan ^(-1)(\tan \theta_1)=\tan ^(-1)(-(2)/(3))=-33.7 \\ \theta_2=\tan ^(-1)(\tan \theta_2)=\tan ^(-1)((1)/(3))=18.4 \end{gathered}`

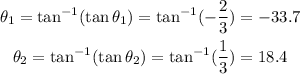
the difference in the angles is the angle between both lines:

18.4 - (33.7) = 18.4 + 33.7 = 52.1

Hence, the angle between the lines is 52.1 degrees