Directions in the picture, i need to do number 30

Question

asked Feb 17, 2023 108k views

1 Answer

Hellfish · Answer 1 · 2023-02-23T02:44:06+0000

Hello there. To solve this question, we'll have to remember some properties about growth or decay functions and how to rewrite expressions.

Given the expression no. 30:

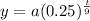
$y=a(0.25)^{(t)/(9)}$

We can rewrite:

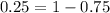
Therefore we have:

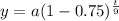
$y=a(1-0.75)^{(t)/(9)}$

In this case, we get r = 0.75, that means a decay rate, because the values will get smaller and smaller as t increases.

The end behavior of this function is getting close to zero when t is sufficiently large.