Question

use casting out nines to determine whether the following calculation could be correct. 730, 963, 709. 28, 100, 923, 561, 398 = 20, 450, 755, 312, 764, 971, 305, 182

Answer 1

Given:

`730,963,709*28,100,923,561,398=20,450,755,312,764,971,305,182`

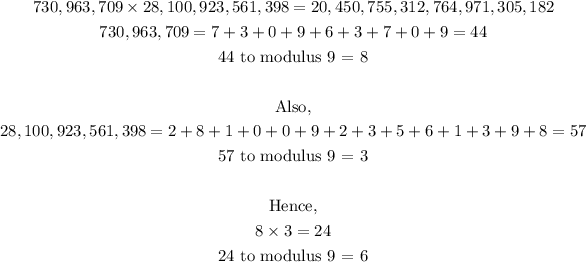
Using casting out nines to determine whether the calculation is correct, means crossing out the 9s or components of 9.

`730,963,709*28,100,923,561,398=20,450,755,312,764,971,305,182`

To know if the calculation is correct, we add the digits of the multiplicand and take the result to modulus 9. Also, we add the digits of the multiplier and take the result to modulus 9.

We then multiply both results together and take to modulus 9 as well.

The result gotten is now compared to the result of the addition of the digits of the result of the calculation in modulus 9.

If both results are equal, then it is a possible correct solution.

Hence,

`\begin{gathered} 730,963,709*28,100,923,561,398=20,450,755,312,764,971,305,182 \\ 730,963,709=7+3+0+9+6+3+7+0+9=44 \\ 44\text{ to modulus 9 = 8} \\ \\ \text{Also,} \\ 28,100,923,561,398=2+8+1+0+0+9+2+3+5+6+1+3+9+8=57 \\ 57\text{ to modulus 9 = 3} \\ \\ \text{Hence,} \\ 8*3=24 \\ 24\text{ to modulus 9 = 6} \end{gathered}`

For the result side;

Since the result from casting out nines gave the same outcome (6 (mod 9)), then the calculation could be correct.