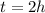The equation of a proportional relationship between two variables x and y with a constant of proportionality k, is:

If y represents the number of toys and x represents the number of hours, substitute the corresponding values of x and y to find the constant of proportionality k. Use, for instance, the fact that Nicholas made 40 toys in 10 hours:
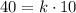
Divide both sides of the equation by 10:
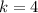
Since Nicholas's toy-making rate is 4 toys per hour, half that rate would be 2 toys per hour. Then, out equation would become:
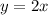
Using the letter "t" for toys instead of y and "h" for hours instead of x, then: