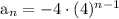Answer:
Step-by-step explanation:
The general exponential form of terms in an exponential sequence is:
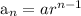
where a is the first term, r is the common ratio and n is the term number
We already have the first term as -4
To get the common ratio, we have to divide subsequent terms as follows:
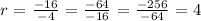
Thus, we have the exponential function as: