Solution:
The question is given below as

Step 1:
Using the following identity below
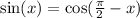
By applying the identity above, we will have
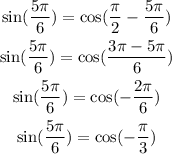
Step 2:
Use the property below
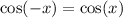
By applying the property above, we will have
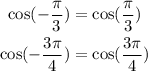
The question above then becomes
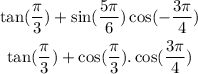
Using the following trivial identities, we will have
![\begin{gathered} \tan \mleft((\pi)/(3)\mright)=√(3) \\ \cos \mleft((\pi)/(3)\mright)=(1)/(2) \\ \cos ((3\pi)/(4))=\frac{-\sqrt[]{2}}{2} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/nvcrjcu079q0wwvhz4vzsgd1pllzzbo9m8.png)
By substituting the above trivial identities, we will have
![\begin{gathered} \tan ((\pi)/(3))+\cos ((\pi)/(3))\text{.}\cos ((3\pi)/(4)) \\ \sqrt[]{3}+(1)/(2)*\frac{-\sqrt[]{2}}{2} \\ =\sqrt[]{3}-\frac{\sqrt[]{2}}{4} \\ =\frac{4\sqrt[]{3}-\sqrt[]{2}}{4} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/jskthw47gfal2ej5isvrcvef8m2h4oszj6.png)
Hence,
The SECOND OPTION is the right answer
![\frac{4\sqrt[]{3}-\sqrt[]{2}}{4}](https://img.qammunity.org/2023/formulas/mathematics/college/f5kg6u009uwyjzt2t6x3s290vdyuhbn1oh.png)