Question

Directions: For each problem - define your variables, set up a system of equations, and solve.1. At the fast food restaurant, four cheeseburgers and five small fries have a total of 2,310 calories. Three cheeseburgers and two small fries have a total of 1,330 calories. How many calories does each item contain?

Answer 1

We know that

• Four cheeseburgers and five small fries have a total of 2,310 calories.

,

• Three cheeseburgers and two small fries have a total of 1,330 calories.

Let's call c cheeseburgers and f small fries.

Based on the given information, we can define the following system.

`\begin{gathered} 4c+5f=2,310 \\ 3c+2f=1,330 \end{gathered}`

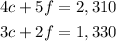
To solve this system, we multiple the first equation by -3/4 to eliminate c and find the value for f

`\begin{gathered} -3c-(15)/(4)f=-(6,930)/(4) \\ 3c+2f=1,330 \end{gathered}`

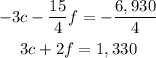
Now, we sum the equations to get one equation with f

`\begin{gathered} (8f-15f)/(4)=(5,320-6,930)/(4) \\ -7f=-1,610 \\ f=(-1,610)/(-7) \\ f=230 \end{gathered}`

This means one small fries has 230 calories.

We use this value to find the calories for a cheeseburger.

`\begin{gathered} 3c+2(230)=1,330 \\ 3c+460=1,330 \\ 3c=1,330-460 \\ 3c=870 \\ c=(870)/(3) \\ c=290 \end{gathered}`

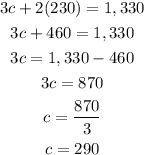
Each cheeseburger has 290 calories.