The equilibrium concentrations are [H2]= 0.126 M, [I2]= 0.526 M and [HI]= 1.748 M.
- First, to calculate the concentrations of all entities at equilibrium, we need to analyze the initial state, the reaction and the equilibrium state:
Remember that thw units of concentrations must be in molarity (M), so we need to convert the 0.50 mol and 0.70 mol.
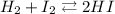
Initial: 1 1.4 0
Reaction: -x -x 2x
Equilibrium: 1-x 1.4-x 2x
- Second, we write the formula for the equilibrium constant (K):

- Now, we solve the quadratic equation with the Bhaskara formula:
In this case, a=42, b=-110.4 and c=64.4.
![\begin{gathered} x=\text{ }\frac{-b\pm\sqrt[]{b^2-4.a.c}}{2.a} \\ x=\text{ }\frac{-(-110.4)\pm\sqrt[]{(-110.4)^2-4.42.(64.4)}}{2.42} \end{gathered}](https://img.qammunity.org/2023/formulas/chemistry/college/l31pugkuo0w9cqskksq3ypsgt92sqcm1h8.png)
We find x1=0.874 and x2=1.755. The correct value for x is x=0.874. We can't use x=1.755 because it is a quantity greater that 1.4.
- Finally, we replace the value of x and solve:
H2 concentration:
[H2]= 1-x
[H2]= 1-0.874
[H2]= 0.126 M
I2 concentration:
[I2]= 1.4-x
[I2]= 1.4-0.874
[I2]= 0.526 M
HI concentration:
[HI]= 2x
[HI]= 2 . 0.874
[HI]= 1.748 M
So, the equilibrium concentrations are [H2]= 0.126 M, [I2]= 0.526 M and [HI]= 1.748 M.