Question

Find the area of ABC if the area of PRT=24 mm^2

Answer 1

Given:

`\triangle ABC\sim\triangle PRT`

As the triangles are similar, the corresponding sides are proportional

`(AC)/(PT)=(BC)/(RT)`

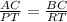
Given: AC = 15, BC = 12 , PT = 6

`\begin{gathered} (15)/(6)=(12)/(RT) \\ \\ RT=(6\cdot12)/(15)=4.8 \end{gathered}`

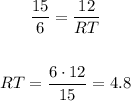
We will find the area of the triangle ABC using the following ratio:

`\frac{Area\triangle\text{ABC}}{Area\triangle\text{PRT}}=(0.5\cdot AC\cdot BC\cdot\sin C)/(0.5\cdot PT\cdot RT\cdot\sin T)`

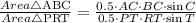
The angle C is congruent to the angle T

So, substitute with the given values:

`\begin{gathered} (Area\triangle ABC)/(24)=(15\cdot12)/(6\cdot4.8)=(180)/(28.8)=6.25 \\ \\ Area\triangle\text{ABC}=24\cdot6.25=150 \end{gathered}`

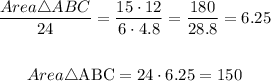
So, the answer will be:

The area of ΔABC = 150 mm²