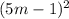Question

Factor 25m squared - 10m + 1

Answer 1

Given:

`25m^2-10m+1`

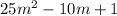
Let's factor the expression.

To factor the expression, first rewrite 5² = 25:

`\begin{gathered} 5^2m^2-10m+1 \\ \\ (5m)^2-10m+1 \end{gathered}`

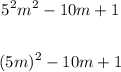
Now, rewrite 1² = 1:

`(5m)^2-10m+1^2`

Now, check that the middle term is two times the product of the first term being squared (5m) and the last term (1).

We have:

2 x (5m x 1) = 10 m

Thus, we have the expression:

`(5m)^2-2*5m*1+1^2`

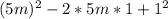
Now, factor using the Perfect Square Trinomial rule:

`a^2-2ab+b^2=(a-b)^2`

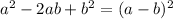
Thus, we have:

`(5m)^2-2*5m*1+1^2=(5m-1)^2`

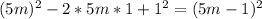
Where:

• a = 5m

,

• b = 1

Therefore, the factored form of the given expression is:

`(5m-1)^2`

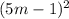
ANSWER:

`(5m-1)^2`