Question

Write an equation, for the line whose slope is -3/2 and passes through the point (4,-7).

Answer 1

The form of the equation of a line is

y = m x + b

m is the slope

b is the y-intercept

Since the slope of the line is -3/2

`m=-(3)/(2)`

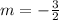
Substitute it in the form of the equation

`y=-(3)/(2)x+b`

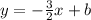
To find b substitute x and y in the equation by the coordinates of a point on the line

Since the line passes through the point (4, -7), then

x = 4 and y = -7

`\begin{gathered} -7=-(3)/(2)(4)\text{ + b} \\ -7=-6+b \end{gathered}`

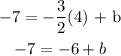
Add 6 to both sides to find b

-7 + 6 = -6 + 6 + b

-1 = b

Substitute the value of b in the equation

`\begin{gathered} y=-(3)/(2)x+(-1) \\ y=-(3)/(2)x-1 \end{gathered}`

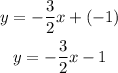
The equation of the line is y = -3/2 x - 1