Question

Determine whether the relation is a functiony=x²+xy

Answer 1

By definition, a relation is a function if each Input value (x-value) has one and only one Output value (y-value). Let's remember that a functions show the relationship between variables

In this case, you have the following relation given in the exercise:

`y=x^(2)+xy​`

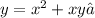
This is a function, because each value of "x" has a value "y". Let's solve for the variable "y", as following:

`\begin{gathered} y-xy=x^2 \\ y(1-x)=x^2 \\ \\ y=(x^2)/(1-x) \end{gathered}`

If you give values to "x", you get the following graph:

Therefore, the relation is a Hyperbolic function.

The answer is: Yes, it is a function.