We have to calculate the sales tax and total sales.
We know the item marked price ($594.47) and the sales tax rate (4.874%).
We can start by calculating the sales tax as the item marked price times the sales tax rate (expressed in decimal form):
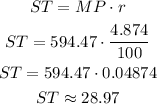
We now can calculate the total sales as the sum of the item marked price and the sales tax:

Answer:
Sales tax = $28.97
Total sales = $623.44