y=4x+64
Step-by-step explanation:
Two lines are perpendicular if the product of their slopes is -1.
Step 1: Find the slope of the line a
Line a passes through the points (1,-3) and (9,-5)
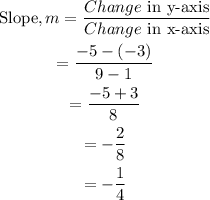
The slope of line a = -1/4
Step 2: Determine the slope of line b.
Let the slope of line b = k
Since the product of the two slopes is -1:
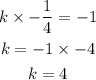
The slope of line b = 4
Step 3: Find the equation of line b.
Line b passes through the point (x1,y1)=(-8,32) and has a slope, m = 4.
Using the slope-point form of the equation of a line:

Substitute the given values
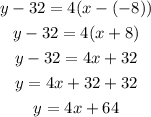
The equation of line b is y=4x+64.