Question

10. Trapezoid PQRS is dilated about point P by a scale factor of 3 to form trapezoid P'Q'R'S'.-QTRPIsWhich statement is NOT true?The measure of angle P'is 3 times the measure of angle P.The perimeter of PQRS is one-third the perimeter of P'O'RS.The length of PQ'is 3 times the length of PO.QR and Q'R' are parallel.

Answer 1

Given:

Trapezoid PQRS is dilated about point P by a scale factor of 3 to form trapezoid P'Q'R'S'.

Required:

We need to find the dilation of Trapezoid PQRS by scale factor 3.

Step-by-step explanation:

Recall that dilation is a type of transformation that enlarges or reduces a figure (called the preimage) to create a new figure.

Dilations preserve angle measure, betweenness of points and collinearity.

Let P be the perimeter of PQRS.

The dilation P'O'R'S' is enlarged by scale factor 3.

The perimeter of the P'O'R'S' is 3 times the perimeter of PQRS.

`The\text{ perimeter of P'Q'R'S' =3p}`

`(1)/(3)(The\text{ perimeter of P'Q'R'S' \rparen=p}`

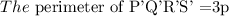
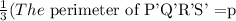
The perimeter of PQRS is one-third of the perimeter of P'O'RS is true.

The length of PQ' is 3 times the length of PQ is true since the scale factor is 3.

QR and Q'R' are parallel is true.

The measure of angle P' is 3 times the measure of angle P is not true.

Dilations preserve angle measure.

Final answer: