Given,
The mass of the car, m₁=9000 kg
The velocity of the car before collision, u₁=8 m/s
The mass of the SUV, m₂=12000 kg
The velocity of the SUV before the collision, u₂=-7 m/s
The velocity of the car after the collision, v₁=-6 m/s
Here we consider that the east is the positive direction and the west is the negative direction, hence all the westward velocities are negative.
The momentum of an object is the product of its velocity and mass.
From the law of conservation of momentum, the total momentum of the car and the SUV before the collision is equal to the total momentum of the car and the SUV after the collision.
Thus,
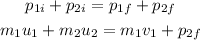
Where:
• p₁i and p₁f are the momenta of the car before and after the collision respectively.
,
• p₂i and p₂f are the momenta of the suv before and after the collision respectively.
On substituting the known values,
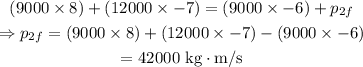
Therefore the momentum of the SUV after the collision is 42000 kg·m/s
The velocity of the SUV after the collision is given by,
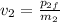
On substituting the known values,
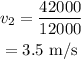
Thus the speed of the SUV after the collision is 3.5 m/s