ANSWER:
A. 840 J
B. 840 J
Explanation:
Given:
Force (F) = 70 N
Mass (m) = 3 kg
Distance (d) = 12 m
A.
The work is given by the following equation:

Work is equal to 840 joules
B.
Initial speed = 0
Final speed = v
We calculate the acceleration just like this:

Knowing this we can calculate the speed:
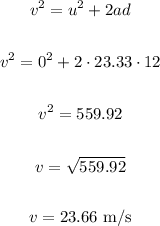
Now we calculate the increase in kinetic energy:
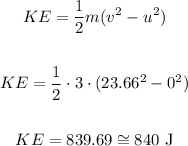
Therefore, the increase in kinetic energy is 840 joules.