Question

It says to Complete each equation so that it has the indicated number of solutions for both

Answer 1

Question 3

We only have to put a different number to one, since this makes that equality is not maintained and the expression has no solution

`\begin{gathered} 3x+1=3x+2 \\ \end{gathered}`

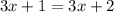
checking

`\begin{gathered} 3x-3x=2-1 \\ 0=1 \end{gathered}`

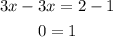
equality is wrong

Question 4

in this case we want the expression to have a solution for any value of x, so equality must be preserved and x must disappear or not matter in the expression

`2x-4=2x-4`

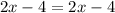
cheking

`\begin{gathered} 2x-2x=-4+4 \\ 0=0 \end{gathered}`

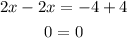
equality is correct and does not interfere, so it can take any value