Question

Calculate the mass of magnesium that is required to produce 27.8 mL of hydroge gas at 26 degrees Celsius and a total pressure of 735 mmHg when collected over water according to the following reaction:Mg(s) + 2fHCl arrow MgCl2 (aq) +H2

Answer 1

Step 1 - Understanding the gas equation

The volume (V), the pressure (p) and the temperature (T) of a gas samples can be related to its number of moles (n) by the gas equation:

`pV=nRT`

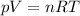
In this equation, R represents the universal gas constant, which may have different values depending on which unities are used.

Let's use L for volume, mmHGg for pressure and K for temperature. In these conditions, R = 62.3 L.mmHg/K.mol.

Step 2 - Calculating how many moles of H2 gas are produced

Now let's set the values in the equation. The exercise states that:

`\begin{gathered} V=27.8ml=0.0278L \\ T=26°C=299K \\ p=735mmHg \end{gathered}`

Substituting in the equation:

`\begin{gathered} 735*0.0278=n*62.3*299 \\ \\ n=(20.4)/(18627.7)=1.1*10^(-3)moles \end{gathered}`

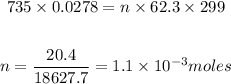
Therefore, 1.1*10^(-3) moles of H2 gas were produced.

Step 3 - Calculating the required mass of Mg

The given equation is:

`Mg_((s))+2HCl_((aq))\rightarrow MgCl_(2(aq))+H_(2(g))`

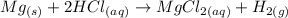
We can see that one mole of Mg produces one mole of H2 gas. This is a fixed proportion. We will always have the same amount of Mg and H2 in moles for this reaction.

Therefore, if 1.1*10^(-3) moles of H2 were produced, it means the same amount of moles of Mg were required: 1.1*10^(-3) moles.

We can convert this value to mass of Mg by multiplying it by its molar mass (24 g/mol)

`m_(Mg)=n* M=1.1*10^(-3)*24=2.64*10^(-2^)g`

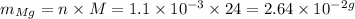
We would need thus 2.64*10^(-2) g of Mg for this reaction.

Answer:

`2.64*10^(-2)g\text{ of Mg}`