Question

A triangle for area 15 square inches has a height that is four less than two times the base. Find the base and the height of the triangle. The base is _ inches and the height is _ inches

Answer 1

Step 1:

Write the formula for the area of a triangle

`\text{Area of a triangle = }\frac{Base\text{ }*\text{ Height}}{2}`

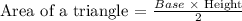
Step 2:

Given data

Let the base = m

height = 2m - 4

Step 3:

Substitute in the formula

`\begin{gathered} \text{Area = }\frac{Base\text{ }*\text{ Height}}{2} \\ 15\text{ = }\frac{m(2m\text{ - 4)}}{2} \\ \text{Cross multiply} \\ m(2m\text{ - 4) = 2}*15 \\ 2m^2\text{ - 4m = 30} \\ \text{Divide both sides of the equation by 2} \\ m^2\text{ - 2m - 15 = 0} \end{gathered}`

Step 4

Factorize the equation to find m.

`\begin{gathered} m^2\text{ - 2m - 15 = 0} \\ m^2\text{ -5m + 3m - 15 = 0} \\ m(m\text{ - 5) + 3(m - 5) = 0} \\ (m\text{ + 3)(m - 5) = 0} \\ m\text{ = -3 or 5} \end{gathered}`

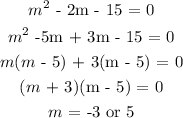
m = 5, the value of m cannot be negative because the measurement of length cannot be negative.

Final answer

Base = 5 inches

Height = 2(5 ) - 4 = 10 - 4 = 6 inches

Base = 5 inches

Height = 6 inches