Question

Identify the holes, vertical asymptotes and horizontal asymptote of each

Answer 1

Since there is no common factor between the numerator and denominator, hence, there is no hole

To get the vertical asymptotes

Equate the denominator to zero

`\begin{gathered} x^2+2x-3=0 \\ (x+1)^2=3+1 \\ (x+1)^2=4 \\ (x+1)=\pm\sqrt[]{4} \\ x+1=\pm2 \\ x=-1-2,-1+2 \\ x=-3,1 \end{gathered}`

The vertical asymptote are x= -3 and x=1

To get the Horizontal asymptote

we will compare the degree of the denominator with that of the numerator

In this case, the degree of the numerator is 2 and that of the denominator is 2.Thus

the degree of the denominator is equal to that of the numerator.

Therefore the vertical asymptote is given by

`\begin{gathered} y=(a)/(b) \\ \text{where } \\ a=\text{ the leading coefficient of the numerator} \\ b=\text{ the leading coefficient of the denominator} \\ In\text{ this case } \\ a=1 \\ b=1 \\ \text{Therefore the vertical asymptote is } \\ (1)/(1)=1 \end{gathered}`

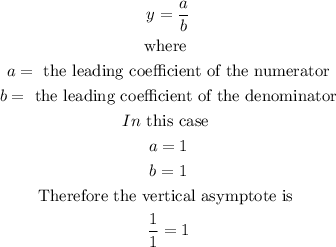
Therefore the vertical asymptote is 1