Question

A train travels 100 km in the same time that a plane covers 450 km. If the speed of the plane is 30 km per hr less than 5 times the speed of the train, find both speeds.

Answer 1

A train travels 100 km in the same time that a plane covers 450 km. If the speed of the plane is 30 km per hr less than 5 times the speed of the train, find both speeds.

Step 1

let

train

distance= 100 km

time= t1

speed=S1

plane

If the speed of the plane is 30 km per hr less than 5 times the speed of the train,in math terms it is,

speed2=(5*S1)-30

time=t1 ( the same time)

distance=450 km

Step 2

the time is given by

`\text{time}=\frac{dis\tan ce}{\text{speed}}`
`\begin{gathered} \text{from train} \\ t=(100)/(S1) \\ \text{from plane} \\ t=(450)/((5\cdot S1)-30) \\ t=t,\text{ so} \\ (100)/(S1)=(450)/((5\cdot S1)-30) \\ 100(5S1-30)=450S1 \\ 500S1-3000=450S1 \\ 500S1-450S1=3000 \\ 50S1=3000 \\ S1=(3000)/(50) \\ S1=60 \end{gathered}`

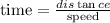
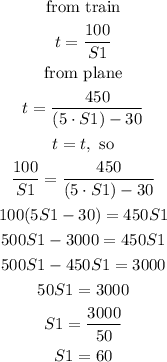
Now, S2

`\begin{gathered} \text{Speed}2=5\cdot s1-30 \\ \text{speed}2=5\cdot60-30 \\ \text{speed}2=300-30 \\ \text{speed}2=270 \end{gathered}`

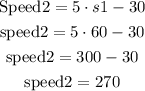
so, the speed of the train is 60 Kph and speed of planes is 270 kph