The given information we have:
The average or mean:

The standard deviation:
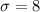
Since Andrew scored 1.25 standard deviations below the mean, the expression to calculate its exam score will be:
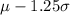
Substituting the mean and the standard deviation:
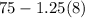
And solving the operations:
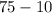
which gives us the following result:
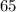
Answer: Andrew's exam score is 65