Imani picked up 40 bushels of apple in 8.2 hours.
And Chelsea picked up 40 bushels of apple in 10.8 hours
Let I be Imani ratio, hence we have

and let C be Chelsea ratio, hence,

Now, let T be the ratio when Chelsea and Imani work together. In this case, we have
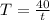
where t is the time for their work.
This means that,
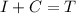
that is, this equality must be fulfilled in order to compare both cases. Hence,

we can factorize 40 in both sides, hence, we have that
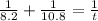
Now, we must solve this equality for t. Therefore, we have
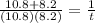
or
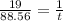
it yields,

Finally, t is equal to
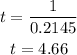
that is, of they work together, they will take 4.66 hours only